Groundbreaking progress in quantum physics: how quantum field theories decay and fission

An international research team around Marcus Sperling, a researcher at the Faculty of Physics, University of Vienna, has sparked interest in the scientific community with pioneering results in quantum physics: In their current study, the researchers reinterpret the Higgs mechanism, which gives elementary particles mass and triggers phase transitions, using the concept of “magnetic quivers.” The work has now been published in the prestigious journal “Physical Review Letters.”

The foundation of Marcus Sperling’s research, which lies at the intersection of physics and mathematics, is Quantum Field Theory (QFT) – a physical-mathematical concept within quantum physics focused on describing particles and their interactions at the subatomic level. Since 2018, he has developed the so-called “magnetic quivers” along with colleagues – a graphical tool that summarizes all the information needed to define a QFT, thus displaying complex interactions between particle fields or other physical quantities clearly and intuitively.

Metaphorical Magnetic Quivers

A quiver consists of directed arrows and nodes. The arrows represent the quantum fields (matter fields), while the nodes represent the interactions – e.g., strong, weak, or electromagnetic – between the fields. The direction of the arrows indicates how the fields are charged under the interactions, e.g., what electric charge the particles carry. Marcus Sperling explains, “The term ‘magnetic’ is also used metaphorically here to point to the unexpected quantum properties that are made visible by these representations. Similar to the spin of an electron, which can be detected through a magnetic field, magnetic quivers reveal certain properties or structures in the QFTs that may not be obvious at first glance.” Thus, they offer a practical way to visualize and analyze complex quantum phenomena, facilitating new insights into the underlying mechanisms of the quantum world.

Supersymmetric QFTs

For the current study, the stable ground states (vacua) – the lowest energy configuration in which no particles or excitations are present – in a variety of “supersymmetric QFTs” were explored. These QFTs, with their simplified space-time symmetry, serve as a laboratory environment, as they resemble real physical systems of subatomic particles but have certain mathematical properties that facilitate calculations. FWF START award winner Sperling said, “Our research deals with the fundamentals of our understanding of physics. Only after we have understood the QFTs in our laboratory environment can we apply these insights to more realistic QFT models.” The concept of magnetic quivers – one of the main research topics of Sperling’s START project at the University of Vienna – was used as a tool to provide a precise geometric description of the new quantum vacua.

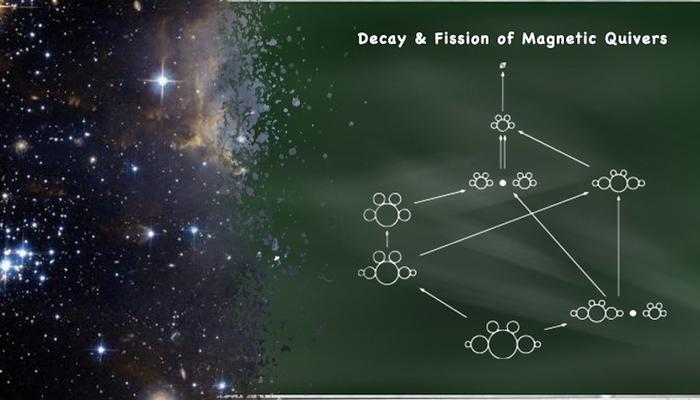
Decay & Fission: Higgs Mechanism Reinterpreted

With calculations based on linear algebra, the research team demonstrated that – analogous to radioactivity in atomic nuclei – a magnetic quiver can decay into a more stable state or fission into two separate quivers. These transformations offer a new understanding of the Higgs mechanism in QFTs, which either decay into simpler QFTs or fission into separate, independent QFTs. Physicist Sperling stated, “The Higgs mechanism explains how elementary particles acquire their mass by interacting with the Higgs field, which permeates the entire universe. Particles interact with this field as they move through space – similar to a swimmer moving through water.” A particle that has no mass usually moves at the speed of light. However, when it interacts with the Higgs field, it “sticks” to this field and becomes sluggish, leading to the manifestation of its mass. The Higgs mechanism is thus a crucial concept for understanding the fundamental building blocks and forces of the universe. Mathematically, the “decay and fission” algorithm is based on the principles of linear algebra and a clear definition of stability. It operates autonomously and requires no external inputs. The results achieved through physics-inspired methods are not only relevant in physics but also in mathematical research: They offer a fundamental and universally valid description of the complex, intertwined structures of the quantum vacua, representing a significant advance in mathematics.